gigalooki.blogg.se - Easy way to derive vector calculus identities

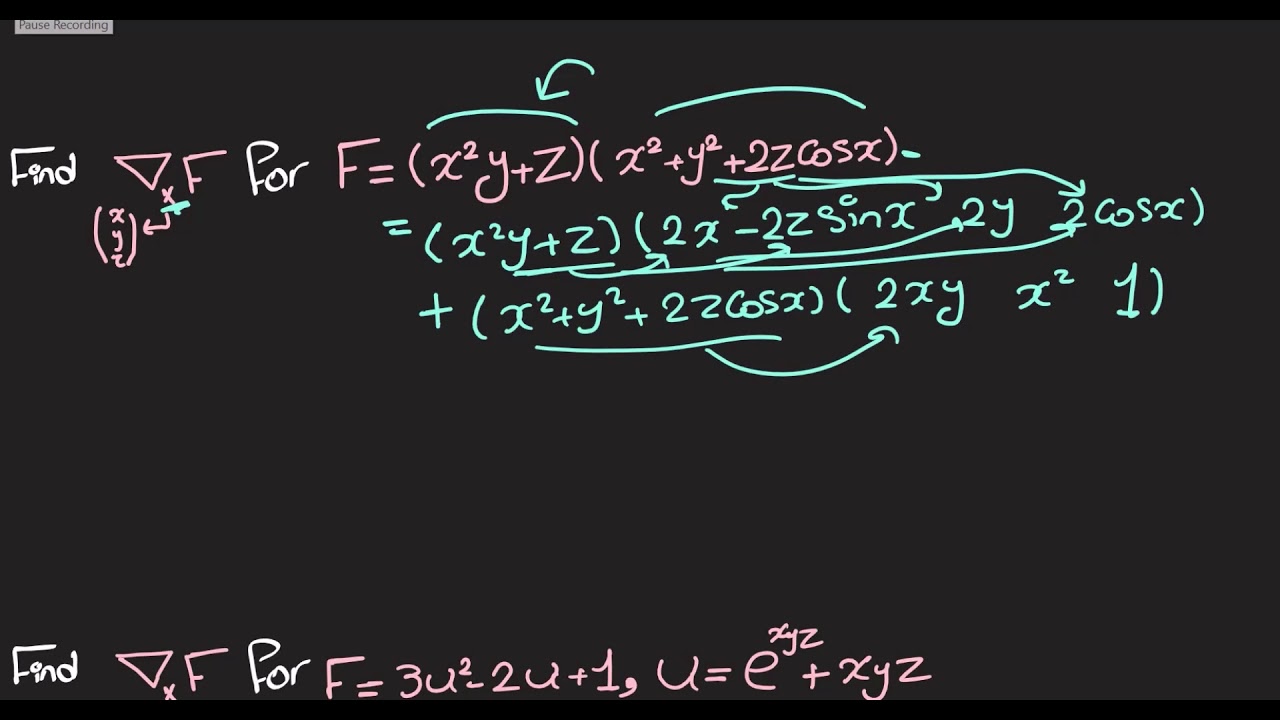
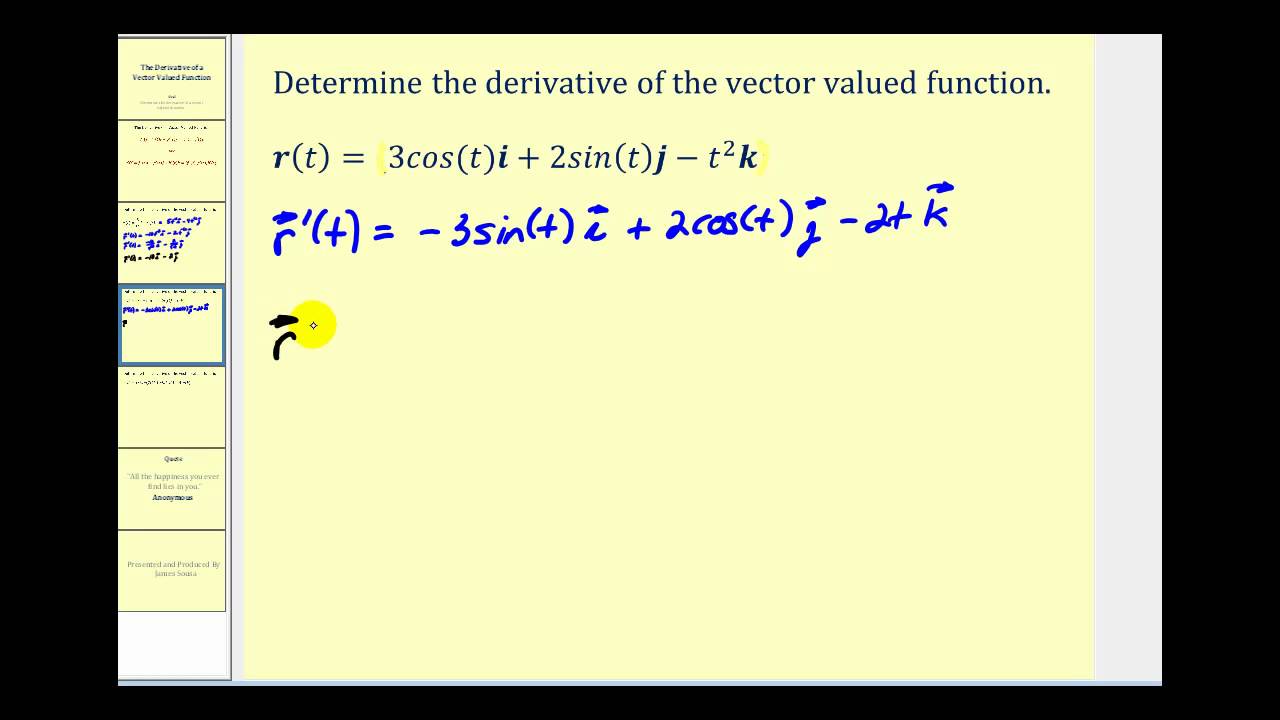
Ques.This may be borderline for this site, but here goes: What you are looking for may be the notion of weak derivative. Therefore, the slope of normal to the given curve is Putting this value in the curve equation. Let the slope of normal line be m1 = −1/m = −1/(1−x)/(y−1) = (1−y)/(1−x)Īs We know that two parallel lines have equal slopes. The slope of the curve here is m = (1−x)/(y−1)

Now, we have to differentiate the curve equation with respect to x. The equation of the curve is x 2 + y 2 = 2x + 2y What is the difference between surface integral and double integral? ( 3 Marks) There are theorems too which relate some unique integrals of these quantities to the other integrals. In particular, there are three types of vector quantities that you can make by using the derivations that are gradient, divergence, and curl. The vector calculus, on the other hand, is related to the aspects of the vector spaces which you treat by using differential and/ or integral calculus. The variables that are involved in both the differential and the integral calculation are generally taken as the real or the complex figures, although the distinct notions of vector, vector spaces, etc. The differential is just a part of the derivations, whereas the integral is a part of the integrals and the integration. Calculus is commonly supposed to be differential calculus and integral calculus. Differential calculus is the part of the calculus that's related to the derivations of the functions.

The Fundamental Theorem of Line Integral states that If F = ∇f and curve C has endpoints A and B, then ∫c F.

A surface integral is an abstraction of various integrals to the integrations over surfaces.

A line integral of a vector field is some function’s integral along a curve.

In the Euclidean space, a domain’s vector field is shown as a vector-valued function that does the comparison of the real number’s n-tuple to each point on the domain.

Vector fields show the distribution of a particular vector to each point in the space’s subset.

It deals with the integration and the differentiation of the vector field in the Euclidean Space of three dimensions.

Vector Calculus can also be called vector analysis.

It is used in Partial differential equation.

Vector Calculus has an important role in various fields such as The list of identities of Vector Calculus are given below for different functions such as Divergence function, Gradient function, Curl function, Degree Two functions and Laplacian function. Read More: Fundamental Theorem of Calculus Vector Calculus Identities If F = ∇f and curve C has endpoints A and B, so We use the fundamental theorems to get the followingį(x, y, z) = P(x, y, z)i + Q(x, y, z)j + R(x, y, z)k Various important formulas for vector calculus are given below: For a particular surface, a scalar field or a vector field can be integrated over a surface. It means that the double integral is related to the line integral.

Read More: Differentiation and Integration FormulaĪ surface integral is an abstraction of various integrals to the integrations over surfaces. Sometimes, a line integral can also be called a curve integral, curvilinear integral or path integral. For example, one can also integrate a scalar-valued function along a curve. The integration can be done with some specific type of functions with vector value along with a curve. In other words, it can be defined as an integral in which the function is calculated along with a curve when it is about to be integrated. Vector calculus is also shown to work in two different forms of integrals known as the line integrals and the surface integrals.Ī line integral of a vector field is some function’s integral along a curve.

Vector analysis is the kind of analysis where we deal with quantities having both the magnitude and the direction. In the Euclidean space, a domain’s vector field is shown as a vector-valued function that does the comparison of the real number’s n-tuple to each point on the domain. Vector fields show the distribution of a particular vector to each point in the space’s subset. It deals with the integration and the differentiation of the vector field in the Euclidean Space of three dimensions. Vector calculus can also be called vector analysis.